OPTOELECTRONICS-Devices and Technologies Vol.2,No.l,p,59-82,June,1987

THE CANCELLATION OF DIFFRACTION IN WAVE FIELDS

Vladimir TAMARI

Tamari 3DD Co.

4 - 2 - 8 - C26, Komazawa, Setagaya-ku, Tokyo 154 Japan

(Received 1 April 1987; accepted for publication 22 May 1987)

Abstract

A new model that describes the diffraction effect is presented, based on the concept of streamlined flow similar to that in fluid or aerodynamics. The field's energy follows curved paths around the diffracting obstacle, unlike the complicated network of straight interfering rays used in the Huygens-Fresnel wavelet concept or Young's explanation of diffraction as scattering of radiation from the aperture rim. Streamline Diffraction Theory (SDT), based on the equation of continuity In Maxwell's formulation for electromagnetic fields, suggests that it is possible to rectify or de-diffract (DD) the field by simple focusing methods near the aperture edge, thereby obtaining superresolution in the focused image, and non-diverging beams such as lasers. These concepts are describe qualitatively and heuristically, and some simple experiments that demonstrate DD are given.

Key Words Diffraction, de-diffraction, resolution, superresolution, waves, lasers.

1. What is diffraction?

Light and other electromagnetic radiation, sound and matter waves, electron and other particle beams and all other wave fields in nature exhibit the effect of diffraction: a wave field is restricted either by the size of the emitting source or by an obstacle placed in its path. As a result, the geometry of the field is changed. First noted by Leonardo da Vinci [1], the effect was called "diffractio" by Grimaldi [2] in a book published in 1665, using the Latin words for "breaking away". Somehow light breaks or bends when it meets the obstacle. When the obstacle is a diffraction grating or a hologram, diffraction can have very useful applications in spectroscopy or holography. Diffraction, however, also prevents the sharp focusing of telescopes, radar systems, scanning microscopes, ultra-sound, and many other imaging instruments. These "diffraction limits" are accepted without question as the ultimate focusing capability of an instrument. Diffraction causes the divergence of laser and particle beams, and again there seems nothing that can be done about this.

Can one cancel such a natural effect? Gravity is another universally present natural effect, and yet it is routinely "cancelled" in satellite environments when the force of gravity is exactly counterbalanced by the centrifugal force and "zero-gravity" is the result. Similarly the gravitational force acting on floating bodies is "cancelled" by the equal and opposite pressure of the surrounding fluid. In this sense, de-diffraction (DD) attempts to counter the diffraction distortion of the field by an equal and opposite bias implemented by special focusing methods.

In Sec. 2 the outline of Streamline Diffraction Theory (SDT) will be presented, in which the effect is treated as a streamlined flow field, analogous to that of a fluid or gas. According to this model, energy spreads in curved pathways, aptly described by the Arabic word for diffraction, in'itaf [or huyud], which implies smooth bending. This fanning out of the field can be observed whenever there is a release of physical restrictions: reeds growing out of the ground; the tail of the peacock; or a crowd emerging from a gate and into an open space. In Sec. 3 it is shown how SDT can conform to the three other basic models currently given to explain diffraction: A) the spread of Huygens-Fresnel wavelets from every part of the aperture; B) the scattering of a boundary wave first proposed by Young; and C) the statistical distribution of photons according to Heisenberg's uncertainty principle. It is to be noted that the first two models were made before Maxwell's time, and are not based an any verified physical phenomena. It will also be shown that quantum effects are now discounted as the cause of optical diffraction. The question of superresolution, or the attempts to focus beyond the diffraction limits, will be treated in Sec. 4, while the DD proposal will be given in Sec. 5. Simple experimental results demonstrating SDT and DD will be presented in Sec. 6, and some of the many applications of DD will be listed in Sec. 7, which concludes this speculative first published paper on de-diffraction.

2. Streamline diffraction theory

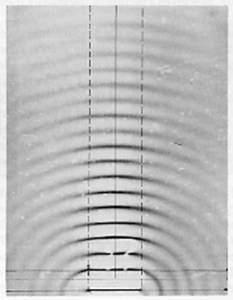
Fig. 1. Water wave diffraction from a plane reflector three wavelengths wide.

Fig. 2. Diagram and photograph of streamline and vortex formation in a fluid

flowing past a sharp obstacle. After S. Goldstein [36].

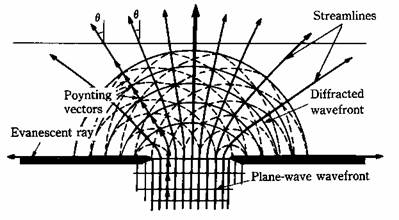
Fig. 3. Diagram of a field diffracted at an aperture. The streamlines show the direction of energy flow. The dashed circles show the interference pattern of two point sources located at the aperture edges and show how scattering from the edge provides an equivalent geometry to streamline formation.

The study of light and that of flowing water have an interesting relationship, and it is not by accident that diffraction is often demonstrated as the spreading of water waves, as in Fig. 1. Al-Hassan Ibn Al-Haytham (Hazen) [3] who first described the orderly passage of light rays through the lens of the eye, also studied the flow of the river Nile and proposed building a dam to control its flow. Leonardo's studies of flowing water clearly illustrate the formation of wakes, a question that will be detailed below, while J.C. Maxwell formulated electromagnetic radiation after first studying streamlined potential flow [4]. This is not surprising, since many different phenomena in nature often obey the same laws. In fluid mechanics or aerodynamics the conservation of matter at a point is expressed by the equation of continuity [5],

(1)

where (ρ) is the density of an incompressible fluid or gas, and (v) is the velocity vector. In electromagnetic theory, Maxwell's equations express the conservation of charge at a point by an identical continuity equation:

(2)

where (ρe) is the charge density, (je) is the current density equal to σ (E + v x B) comprising the conductivity (σ), the electric and magnetic components of the field, and the velocity vector. Born and Wolf remarked: "A description of propagation of light in terms of a hydrodynamical model is often helpful in connection with scalar diffraction fields, as it gives a picture of the energy transport in a simple and graphic manner" [6].

The equation of continuity provides the fundamental theoretical basis of SDT because it describes the direction of the velocity vector. When the field is limited either at the source or by an obstacle in its path, it compensates for the interruption of flow by a systematic distortion. Figure 1 shows that diffraction is nothing more than a topological transformation of the field, whereby the straight ripples become ellipsoidal as soon as the aperture plane is passed, and thereafter expand regularly as parallel ripples until infinity. It will be seen in Sec. 5 how the essence of DD is to prevent the distortion of the first wavefront after the aperture plane, and after that the field does not encounter any other obstacle and will continue undistorted.

The wavefronts of a field are the surfaces of equal phase, and they are familiar in optical terminology. In a flowing fluid or gas, however, the energy density can be constant in time and no waves produced. Energy flows along streamlines, and the velocity vector is always tangent to these streamlines. By definition, there is no energy flow between adjacent streamlines. Typical streamlines in fluid flow are shown in Fig. 2, for the case where the flow bends around an obstacle, at the same time causing the formation of a vortex. The streamline function (Ψ ) is given by:

dΨ = -vd x + udy , (3)

where u,v are the velocity components for x and y. For incompressible flow ∂ρ ⁄ ∂t = 0 along any given streamline, and the equation of continuity in terms of the streamlines becomes

(4)

which can be differentiated in either order, automatically satisfying the continuity Eq. [7]. Streamlines describe smooth or laminar flow, but it often happens that the field rotates around itself (eddy or vortex) or moves randomly in regions of turbulent flow. It is useful to remember these concepts when dealing with the diffracted field.

According to electromagnetic theory the direction of energy flow from a closed region is given by the Poynting vector

S (E x B ) (5)

but when the field experiences turbulence or vortices are formed near the diffracting edge or in the focal region, S cannot be defined locally. But for our purposes here it is sufficient to say that S defines the velocity vector, is tangent to the streamlines, and is normal to the wavefronts measured one wavelength apart along the streamlines. Both the streamlines and the wavefronts are thus equivalent descriptions of the same field, as shown schematically in Fig. 3. In fluid flow, Fig. 3 is known as a flow net, made up of the streamlines and the orthogonal equipotential lines of constant velocity potential Φ. A similar pattern occurs in statics, and electrical fields are used to model fluid flow [37].

In the electromagnetic case, Braunbek and Laukien [8], plotted an H-polarized plane wave diffracted by an infinitely thin perfectly conducting half-plane, based on Sommerfeld's well-known solution of this diffraction case [9], shown in Fig. 4. The interesting thing about Sommerfeld's solution is that mathematically it yields a cylindrical wave scattered by the edge, interfering with a geometrical undiffracted wave. In physical terms, however, there is no trace of this cylindrical wave, and the energy flow follows the streamlined pattern.

While the wavefronts of Figs. 3. and 4 are generally parabolic, the streamlines are hyperbolic, inclined at angles θ to the normal direction. The evanescent ray (θ = 90°) is parallel to the diffracting obstacle, and its existence was experimentally proven by microwave tests [30]. The central streamline of a symmetrical aperture lies on the optical axis (θ = 90°) for a normally incident wave. Away from the edge, the streamlines are bent less and less, so that it can be said that most of the diffraction distortion is initiated within a wavelength from the edge. Fig. 5 shows the same streamlines as Fig. 4, but this time superposed with the intensity patterns of the field. It might seem paradoxical that while the energy is moving behind the aperture, the intensity pattern radiates in the opposite direction, starting from the edge and spreading towards the open portions of the aperture, as in Fig. 6 (a). This can be explained as follows:

As the field bends it becomes warped (just as a piece of cloth develops folds when bent) and a standing intensity pattern is created. This is exactly equivalent to the wake created by a fluid flowing through a gate. The effect can be easily demonstrated with water flowing rapidly past a fixed plate. The stationary intensity waves are created, but the streamlines (shown by the path taken by soap-suds or floating particles in the water) go across these waves smoothly, as illustrated in Fig. 6 (b). That is how the well-known intensity pattern caused by diffraction shown in Fig. 7 is created

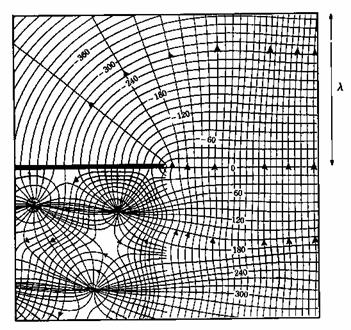
Fig. 4. The streamlines (arrows) and the wavefronts normal to them of an H-polarized field diffracted by an infinite half-plane. Note turbulence and vortex formation

in the reflected portion of the field (bottom left).

Fig. 5. The streamlines (arrows) and amplitude contours of the same field shown in Fig. 4.

Figs. 4 and 5 are adapted from Braunbek and Laukien [8].

Fig. 6. (a) Intensity contours forming the wake of a diffracted field. The streamlines cross these contours at various angles. (b) The streamlines of the field carry energy in one direction,

but form standing intensity contours in the other direction.

Fig. 7. The amplitude of a diffracted plane wave three wavelengths behind an aperture

compare with Fig. 6 (a). The shaded part is the shadow region

Again, the standing intensity pattern seems to radiate from the diffracting edge, but there is no physical basis for saying that energy radiates from the edge, since energy cannot move across streamlines. Scattering from the edge, while a useful mathematical model, simply does not occur from a diffracting obstacle.

Another confusing question raised by SDT, that of interference, must now be examined. Two or more waves can interfere linearly; diffraction is currently almost universally treated as being the result of interfering straight rays emerging from the aperture. Yet in the streamlined flow described above, the energy propagates along separate streamlines, and as in Fig. 1 the diffracted wavefronts absolutely never meet and there is no interference of any kind! This recalls Dirac's statement that "each photon interferes only with itself " [10]. When the diffracted field is focused, the streamlines and wavefronts do merge, but it is the merging of the intensity patterns that causes the typical focused spread functions, and not just the interference of an infinite number of rays integrated at an observation point. This is not to deny of course that waves can interfere, but to stress that physically, the relationship between diffraction and interference is quite different from current models. Figure 8 shows the image in the focal plane of a circular aperture diffracting a focused plane wave, known as the Airy function. Most of the energy is focused into the central bright disc having angular radius

(6)

where D is the diameter of the aperture and λ the wavelength of the field. Eq. (6) is known as the Rayleigh limit of angular resolution of an optical instrument, since if two Airy functions are superposed at a distance of less than RA, the two spread functions will merge into one point, defining the "diffraction limits" of the instrument's power of resolution.

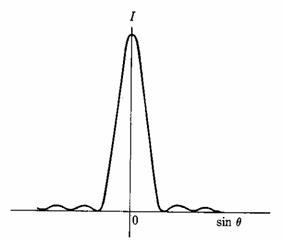
Fig. 8. Airy function is the image of a distant point object focused by a circular lens or mirror.

Fig. 9. Photograph of focused and diffracted water waves produced by a parabolic reflector.

The Airy disc is surrounded by weak rings of decreasing intensity spreading out to infinity in all directions. The wavefronts of the focused field are shown in Fig. 9, but only the bright central intensity pattern can be seen, while the standing waves that cause the ring system are not visible in this photograph. It is interesting to compare the shape of the wavefronts of Figs. 1 and 9. Focusing simply creates phase retardation in a diffracted pattern, making a dent the shape of the reflector or lens, and this pattern is transmitted from wavefront to wavefront, but without affecting the "lost" energy spreading out away from the geometrical focus.

Having explained the diffraction phenomena in terms of SDT, we must now show that this model agrees with the highly developed and successful theories currently in use.

3. Other diffraction theories

SDT differs from currently accepted theories of diffraction in two related ways. The first is the question of interference mentioned above, and the second is that in the currently accepted theories the "energy propagates along straight rays, while in SDT the streamlines are curved. This curvature of course has nothing to do with relativistic gravitational attraction that causes light to bend in the region of a massive galaxy, where the deflection is given by α =2.9-28M/b where M is the mass of the object and b the distance to the ray of light*. In general, diffraction theories may be classified in the following categories:

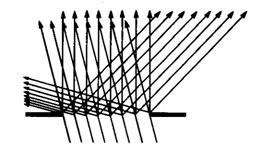
Fig. 10. Huygens-Fresnel wavelet formation (HFP).

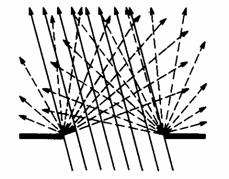
Fig. 11. Boundary diffraction waves (dashed lines) and the geometrical rays (solid lines) (BDW).

Fig. 12. Streamline formation (SDT).

Fig.13 Huygens-Fresnel-Principle (HFP), Boundary Diffraction Wave(BDW) theory,

and Streamline Diffraction Theory (SDT) all explain the same propagation vector S,

but only SDT is physically realistic.

3.1 Theories based on Huygens-Fresnel wavelets concept Many rigorous diffraction theories currently in use are based on Huygens' principle [11] that a wavefront is formed when each element of the preceding wavefront emits a spherical wavelet. This idea was adapted by Fresnel, and the Huygens-Fresnel Principle (HFP) was the basis of the first successful model to describe diffraction phenomena [12]. Kirchhoff refined this concept using potential theory [13], but the basic concept remained unchanged: diffraction is caused by the interference of an infinite number of rays emitted from every part of the aperture, as in Fig. 10. Each point emits most strongly in the forward direction, but the rays lose their amplitude by a factor of (1 + cosθ)/2, known as the inclination factor. It was later shown that Fourier analysis can be used to describe this model, whereby the intensity of the field at a certain point is the Fourier transform of the aperture function [24]. These models based on HFP can accurately predict the field if the point of observation and D are both much larger than A. Yet it is widely accepted that there is no physical basis for HFP: photons simply do not explode into photonettes and spread in all directions the moment they cross an imaginary line in the aperture plane.

3.2 Theories based on Young's boundary wave concept Another group of successful diffraction models is based on Thomas Young's famous double slit interference experiment, when the waves leaving the slits interfere to form bright and dark fringes [14]. Young suggested that the edge of the diffracting aperture is a source of a scattered boundary diffraction wave (BDW) which interferes with the original so-called geometrical wave, yielding the final field, as in Fig. 11. Sommerfeld [9], starting out with Maxwell's equations, showed that a cylindrical wave from the edge can emerge from the mathematical treatment involved. Rubinowics also demonstrated that HFP and BDW are equivalent [15], while more recently Keller put forward his Geometrical Theory of Diffraction [16], based on BDW, which was extended by Miyamoto and Wolf [17]. Again, these mathematical models are not based on any demonstrable physical principles: apart from the impossibility of energy crossing from one streamline to another, photons of the 2πR region of the rim of a circular aperture must interfere with the photons emitted by the entire vπ2 area of the aperture which is physically impossible in terms of the available energy. The great interest in the relationship between the aperture and the image planes must have distracted from the study of the actual streamlined path taken by the photons, as in Fig. 12.

Since HFP, BDW and SDT all describe the same phenomena, they must all be mathematically related, but it is beyond the scope of this paper to develop a complete proof. Instead, Fig. 13 shows how the three models can account for the formation of the same Poynting vector normal to the elliptical wavefront. As S rotates around the wavefront at different 6 it gives the various Fourier orders of the field.

3.3 Diffraction theory based on quantum effects With the emergence of Heisenberg's uncertainty principle, it was tempting to try and explain optical diffraction and interference as just a statistical probability distribution of the field. While such an explanation seems to fit the observed facts fortuitously [18], "attempts to find quantum effects in the physical optical fields of diffraction and interference-"were convincingly negative "[19]. Marcuse, however finds that quantum effects do provide an ultimate limit to the resolution by about an order of magnitude less than the Rayleigh limits [20]. This question was addressed during a discussion of Toraldo di Francia's superresolution proposal, and the conclusion was that "the only correct quantum electrodynamical version of [Heisenberg's] principle imposes no relevant restrictions on resolving power to begin with" [21], which seems to confirm that diffraction is basically a problem in classical and not quantum optics*.

4. Superresolution

An ideal imaging instrument gives a perfect image for any object [22], and theoretically all the relevant information to reconstruct a finite object can be found on the aperture plane, since its Fourier transform on the aperture is an analytic function [23]. The moment the radiation emerges from the aperture, however, it experiences diffraction spreading out, and the resulting point spread function (PSF) is not a point or circle one wavelength in diameter, but rather the Airy function for Fraunhoffer (far-field) diffraction, and a system of luminous rings for Fresnel (near-field) diffraction from a circular aperture. Numerous studies have shown that this diffraction in the presence of noise makes it impossible to obtain resolution better than that of the Rayleigh limits [32]-[34].

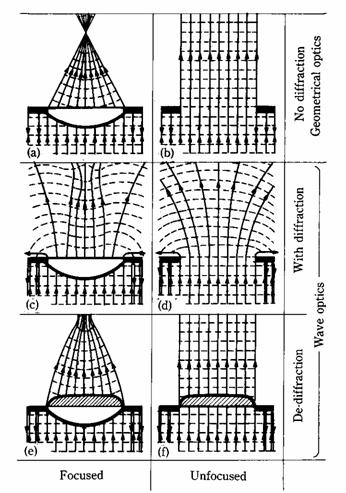
Fig. 14. Diagrams showing the focused and unfocused fields passing an aperture.

De-diffraction lenses are shaded in (e) and (f).

Why is the focus not sharp? In terms of SDT the answer is simple and direct: the field experiences a topological transformation, mostly near the edge, which continues to spread out as the field propagates. Figs. 14 (a), (b) show how, in the absence of diffraction, an ideal imaging instrument should focus a field or transmit a beam without divergence. In real life, however, the focused field (c) spreads out in all directions (compare with Fig. 9). An open aperture, such as that of a laser, experiences the well-known divergence of its Gaussian profile by an angle of about (λ/D), but the total energy spreads through 180° (compare Fig. 1 with Fig. 14 (d)). The portions of the wavefront that can never reach the focal point are the lost Fourier components, which can be thought of as containing the high-frequency spatial information of the object function [24]. The resulting image will appear out of focus, since it will be a convolution of the object function with the PSF. Despite all these theoretical difficulties, it is so important to obtain the best resolution possible that various attempts at superresolution were made.

The work of Schelkunoff to increase the directivity (supergain) of microwave antenna arrays [25] can be considered the pioneering attempt at superresolution. G. Toraldo di Francia adapted these ideas to optical apertures, suggesting that the normally open aperture of a lens or mirror can be divided into a chosen number N of annular zones changing the amplitude and phase of the incoming radiation [21]. Acting somewhat like a zone plate, this superresolving filter enables the field to be focused to an arbitrarily thin central maximum, surrounded by a dark ring free of radiation. It was found, however, that increasing the number N of the zones, would make the central peak fainter as well as thinner, concentrating the radiation into the surrounding rings, and creating a giant side lobe [26]. Freiden, working on the parallel problem of image restoration, has proposed one superresolving filter function, shown in Fig. 15 (a), (b), together with the resulting spread function [27]. Because of the very limited size of the dark field surrounding the faint maximum, together with the great difficulties of manufacturing the required phase and amplitude changes, these filters seemed of little practical value in solving the problem of resolution [28].

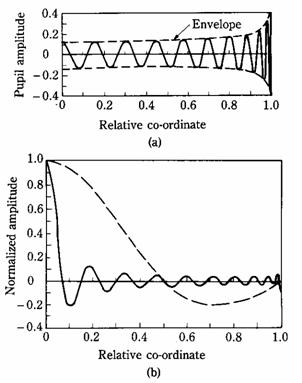
Fig. 15. (a) Complex amplitude distribution of a superresolving filter. The dashed lines show the limiting case when N=∞ and there is a continuous change of phase. The solid line gives the case for N= 40.

(b) The point spread function for the filter shown in (a) when N=40 (solid line), and of an uncoated aperture of the same size (dashed line). After Freiden [27].

What would happen if N is made infinite? As N approaches infinity, all the radiation will be concentrated in one giant side-lobe at θ= 90°. But with N infinite, the aperture can be regarded as completely open, and if so, the phase changes will consist of the smooth envelope of the phase and amplitude modulations shown in Fig. 15 (a). In that case, wouldn't the image function experience an inversion and the radiation become continuously focused to a very thin and very bright central maximum with no side lobes at all? This is the question which was posed by the author [29], not at first in terms of such a filter, but in an attempt to re-focus the outwardly diffracted streamlines, as will be discussed in the following section.

5. De-diffraction

The streamline diffraction pattern of Fig. 3 is reminiscent of the rays from a distant object diverged by a concave lens. The central ray is transmitted without change, but the rays become more and more bent as the edge is approached, where the evanescent ray is formed.

.It is well known that by adding a convex lens to a concave lens of equal diameter, curvature and index of refraction, the resulting plate with parallel sides will transmit the rays unchanged. Why not think of the whole diffraction process as the action of an imaginary, generally concave "diffraction lens"? To cancel the divergence of the field produced by this "lens", its convex equivalent, a de-diffracting DD lens, is added to the open aperture. The resulting pair would then consist of an imaginary, generally concave "diffraction lens" and an actual DD lens; their combined action should cancel the diffraction effect, as in Fig. 16 (a), (b), and Fig. 14 (e), (f).

Imaginary “diffraction lens” Real de-diffraction lens

(a) (b)

Fig. 16. (a) Diffraction can be conceived of as the action of an imaginary "diffraction lens"

(b) The cancellation of diffraction can be conceived as the action of a compound lens

made up of the imaginary lens (a) and a real DD lens

Intuitively this refocusing would consist of trying to prevent a plane wavefront, arriving normally at the aperture, from becoming the elliptical wavefront one wavelength away. Once the wave passes the aperture without distortion, no other obstacle will be in its path, and it will not diffract anew. In more analytical terms [31], and referring to Fig. 17, we can describe the process as follows.

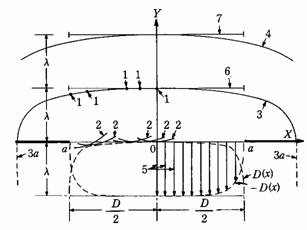
Fig. 17. Geometry of de-diffraction from an aperture.

An aperture a-a is centered around the origin in an infinite thin screen found on the X-axis, with the plane wave arriving from below with wavefronts (λ) apart from and parallel to the X-axis. The portion a-a of this wavefront experiences the diffraction transformation, and spreads out to become the wavefront (3). It is obvious that the screen's presence causes this distortion.

Let us now heuristically remove the screen completely and ask: "What wavefront D(x) between a-a can create (3) in free space?" Using the principle of optical reversibility, D(x) can be thought of as the wave created when (3) moves backwards towards the origin. D(x) is thus defined by the envelope of Huygens wavelets (2) of radius (A) drawn from centers (1) evenly distributed all along (3). In the -y regions these centers can be drawn from the lines (3a) shown in the figure.

Having obtained D(x), we can say that

i) (The diffracting screen) transforms a-a into (3)

ii) D(x) transforms a-a into (3)

iii) Therefore D (x) is equivalent to (The diffracting screen)

iv) D(x) - D(x) = a-a and not (3)

Similarly,

vi) (The diffraction screen) - D(x)=a-a and not (3).

In other words step (vi) says that introducing a phase retardation -D(x) will exactly oppose the diffraction effect. The process of focusing can be thought of as a phase retardation, so that (DD) is an equal and opposite refocusing of the field to oppose the diffraction "focusing" effect. Once -D(x) passes the aperture a truncated plane wave (6) is created. This "liberated" wave, following the law of conservation of momentum, moves parallel to itself and does not experience any further sideways spread: DD enables the creation of a new type of undiffracted wave not found in nature. From purely geometrical considerations, D(x) must be of the form of the +x portion of a Lame oval or so-called superellipse [35], [38]:

(7)

giving

(8)

In real optical systems, DD can be accomplished by first accurately determining the shape of the first diffracted wavefront (3), and then trying to fit the best possible D (x) to suit the geometry of the situation, which will change not only according to the physical structure of the aperture, but also with such parameters as the field's state of polarization, coherence, and intensity distribution. Computer analysis for current flow is recommended for finding the wavefront (3).

Once D (x) is found it can be implemented as a phase change, which means a corresponding physical change in the shape of the reflector or lens, so that a plane wave entering the system will arrive at the aperture as - D(x) and thus emerge undiffracted. It is to be noted that introducing - D(x) at the aperture allows a field passing the aperture to emerge without distortion in a truncated form. In open apertures such as those of a laser, the emerging beam should propagate without divergence. If focusing is required, however, D (x) can be simply subtracted from the phase change normally required for geometrical focusing.

For example, the addition of the DD lens to the lens and aperture of Fig. 14 (c), (d) will yield a DD focused field e, and a non-diverging beam/. From Eq. (8) it can be seen that D(x) has a maximum thickness of A; so for a DD laser, the DD lens will be no more than a very thin film with a very accurate profile near the edge. In a focused wavefront, the image function should be no more than A in width, giving a much brighter and thinner central maximum than the Airy disc, with no ring system, having an angular radius Rs=λ/(2F) where F is the focal length or image distance. The resulting gain in resolution will be, from Eq. (6):

(9)

which can be considerable for systems with a large/number FID. Superresolution would change the design of imaging instruments so that mere size of a lens or reflector would not have any effect on the resolution, but only on the energy-gathering capabilities of the system. Large focal lengths to improve the gain G might be needed. DD should not be confused with the concept of apodization [39]. In the latter case only the amplitude of the field near the edge is changed, not its phase. This causes the rings of the Airy pattern to disappear, but without reducing the width of the central disc.

6. De-diffraction experiments

Several experiments were performed with ultrasound radiation, with water waves in a ripple tank and by vision through a thin slit to verify the basic premises of SDT and DD. While these experiments provide preliminary proof of the correctness of this new approach, they must be repeated with electromagnetic fields using much more sophisticated equipment and mathematical treatment than are used here.

Nevertheless the author feels confident that the theoretical analysis above has been verified by the following and other experiments.

Fig. 18. Intensity profiles of a reflected ultrasound field. A plane reflector (A) yields an spreadfunction(D)

The superelliptical reflector (B) yields a sharper and more intense spread function (C). Both reflectors were 61 mm wide, λ=8.3 mm and the images were sensed at a distance of 63 mm from the reflector.

Fig. 19. Streamline formation of an ultrasound field reflected by a parabola. The geometrical (undiffracted) rays are shown in solid lines, and the width of thecalculated spread function

shown by the small arrow at the focus.

6.1 DD of ultrasound waves A point source of ultrasound radiation A =8.3mm was used to emit a field reflected from a plane reflector, and then separately from a two-dimensional reflector of the same width, but with a super-elliptical profile D(x). The resulting amplitudes were measured along a line perpendicular to the .y-axis, and the results plotted in Fig. 18 (c),(d). It is clear that by merely curving the edges of the reflector inwards by about a wavelength, considerable concentration and increased intensity in the reflected radiation is achieved. It might be objected that this is nothing but an ordinary focusing of the field, comparable to the correction of aberrations. But that is exactly what DD is: just a refocusing of the field near the edge. In other ultrasound experiments, a directionally-sensitive sensor was used, and the focused field plotted, confirming the presence of the streamline pattern, as shown in Fig. 19, the field of a parabolic reflector.

Fig. 20. Photograph of unfocused de-diffracted water waves. The superelliptical reflector emitted energy mostly in the forward direction. Compare with Fig. 1 for a normally diffracted field from an aperture of the same size.

Fig. 21. Photograph of de-diffracted focused water waves. The superparabolical reflector (the figure is a parabola added to a superellipse) concentrated the energy towards the focus. Compare with Fig. 9 for a normally diffracted field from a parabolic reflector of the same size.

6.2 Water wave experiments. Diffraction of water waves was simulated by a vibrating plate in a ripple tank illuminated with a light source flickering at the same frequency, effectually freezing the resulting pattern for observation and photography. By varying the shape of the plate, the diffracted wavefronts from an open aperture (flat plate) or a parabola or superparabola (a parabola function added to D(x)) can be easily obtained. It was instructive to observe the effect of real-time changes in the reflector shape on the resulting wavefront, which mimicked the reflector's shape and transmitted this shape as it propagated forward. Thus, when the edges are bent inwards, the whole system of succeeding wavefronts up to the focal point will be "tucked in". A reflector with D=32 mm and A = 10.5 mm of shape D(x) was successfully used to create a DD wave shown in Fig. 20, which should be compared with Fig. 1 of a flat plate of the same width. In the latter case the energy spreads out over 180° but in the DD beam the energy is concentrated within about 30°, with no disturbance on either side, convincingly proving the success of de-diffraction. With better procedures, there seems to be no reason why the wave of Fig. 20 should not be focused only in the forward direction, simulating the field of a DD laser, as in Fig. 14 (f). Note in Fig. 20 how the first wavefront is truncated on either side, while that of Fig. 1 is stretched over the width of the obstacle. The simulation of DD for a focused field, Fig. 21, was less successful, since a small FID ratio had to be used, yielding a small gain according to Eq. (8), and also because of difficulties in manufacturing a smooth two-dimensional superparabola. Nevertheless, even with the simple equipment used in this experiment, some improvement in the focus can be observed, and, equally important, there is a significant reduction of energy in the shadow regions.

6.3 Vision through a pinhole or slit According to SDT, an aperture acts like a converging lens as in Fig. 16 (a). This can be confirmed experimentally when a person with myopic (nearsighted) vision looks through a pinhole of about 1 mm diameter made in a sheet of black paper. At the center of the hole the acuity is improved noticeably. The pinhole acts like a converging eyeglass lens to correct vision.

Similarly, a slit of about 1 mm width acts like a cylindrical converging lens. A person who has astigmatism can correct his or her vision by looking through the slit inclined at the same angle as the astigmatic distortion of the eye.

In this regard one speculates whether human or animal eyes have any DD mechanism. In "soft-edged" lasers with a curved (streamlined) edge, the beam concentration is enhanced [40]. Is there a similar effect at the rim of the eye's iris?

Fig. 22. (a) An example of a DD unfocused superelliptical reflector with a profile

D(x) for an aperture D and wavelength λ=D/5.

(b)An example of a DD focused superparabolic reflector whose profile

is a parabola P (x) having a focal length f =D, added to the superellipse

D(x), for an aperture D and wavelength λ=D/5.

7. Conclusions

A new outline streamline diffraction theory has been proposed which shows that, based on Maxwell's equations, a field experiences a topological transformation when it encounters an obstacle, or is restricted at the source. On the basis of SDT it was suggested that a systematic refocusing of the field through a phase-change - D(x) will exactly counter the diffraction effect, yielding a DD field which can be transmitted in the forward direction or focused to a superresolved point. The preliminary treatment given here will have to be developed with more rigor.

An apparent anomaly raised by SDT is the slowing down of the field as it bends. This means that the original or mainstream velocity is c along unbent pathways, but there is a slowing down (a blue shift?) as the streamlines bend more severely. This is confirmed in Fig. 4. Experimental confirmation of this effect will complete the analogy between the diffracted scalar electromagnetic field and the hydrodynamical model.

The success of DD means the improvement of a wide range of imaging instruments such as cameras, binoculars, telescopes, scanning microscopes, microwave antennas, radio telescopes, phase-array radar, ultrasound imaging, sonar and other acoustical systems, the prevention of divergence .in lasers, electron and particle beams and others. DD concepts can even be applied to sea-walls to allow small boats to pass near these walls without bending away from their intended path. The effect of using DD concepts in the design of integrated optical devices, optical fiber connections and optical memories and computers remains to be studied.

Acknowledgments

Thanks are due to Captain Masato Kobori for building the ultrasound equipment used in the experiments, and to various reviewers who have been helpful in correcting some aspects of SDT, particularly Dr. Nabil Kassis of the Physics department of Birzeit University in occupied Palestine.

*After this paper was published, the author reversed the opinion stated In Sec.3 above, that the streamline curvature has nothing to do with Einstein’s idea of light bending in a gravitational field. SeeV.F.Tamari “Diffraction to De-Diffraction” (1993 published 2003 at http://jp.arxiv.org/abs/physics/0303073 ). The opinion stated in Sec. 3.3 that quantum and diffraction effects are not related was also reversed. This is treated in detail in V.F.Tamari’s “United Dipole Field” (1993, published in 2003 at http://jp.arxiv.org/abs/physics/0303082 ).

References

[ 1 ] M. Born and E. Wolf, Principles of Optics, 6th ed., Pergamon, Oxford (1980) 370.

[2] F. Grimaldi, Physico-Mathesis de Lumine, Bologna (1665).

[3] H. Al-Haytham, Kitab Al-Manazir, Cairo (late 10th c.).

[4] J. Maxwell, Trans. Roy. Soc. Edinb., 26, 1-40 (1869-1872) 161-207.

[5] G. Campbell, Foundations of Fluid Flow Theory, Addison Wesley, Reading, Mass(1973)167.

[6] M. Born and E. Wolf, Principles of Optics, 6th ed., Pergamon, Oxford (1980) 10.

[7] R. Daugherty, J. Franzini, and E. Finnemore, Fluid Mechanics with Engineering Applications, 8th ed.,

McGraw-Hill, New York (1985) 71, 134.

[8] W. Braunbek and G. Laukien, Optik, 9 (1952) 174-179, reprinted in M. Born and E. Wolf, Principles

of Optics, 6th ed., Pergamon Press, Oxford (1980) 576-577.

[9] A. Sommerfeld, Math, Ann., 47 (1896) 317

[10] P. Dirac, Quantum Mechanics, Clarendon, Oxford (1930) 9.

[11] C. Huygens, Traite de la Lumiere, Leyden (1690).

[12] A. Fresnel, Ann. Chim. et Phys. (2), 1 (1816) 239.

[13] G. Kirchhoff, Berl. Ber. (1882) 641.

[14] T. Young, Phil. Trans. Roy. Soc., London xcii (1802) 12,387.

[15] A. Rubinowics, Progress in Optics, 4, North Holland, 199.

[16] J. Keller, J. Appl. Phys., 28 (1957) 426-444.

[17] E. Miyamoto and E. Wolf, J. Opt. Soc. Am. (1962) 615-625, 626-637.

[18] R. Glauber, in Quantum Optics, edited by S. Kay and A. Maitland, Academic Press,

London (1970) 58.

[19] R. Sillito, in Quantum Optics, edited by S. Kay and A. Maitland, Academic Press, London (1970) 2.

[20] D. Marcuse, Light Transmission Optics, Bell Lab. Ser. Van Nostrand, New York (1972)129.

[21] G. Toraldo Di Francia, Suppl. Nuovo Cimento, IX, Series IX (1952) 438.

[22] J. Maxwell, Quart. J. Pure Appl. Math., 2 (1858) 233

[23] H. Wolter, Progress in Optics, edited by E. Wolf, North Holland,

Amsterdam (1961)157.

[24] J. Goodman, Introduction to Fourier Optics, McGraw-Hill, New York (1968).

[25] S. Schelkunoff, Bell Syst. Techn. Journ., 22 (1943) 80.

[26] G. Toraldo Di Francia, Revista del Nuovo Cimento, Serie I, 1 (1969), Num. Spec. 460-484.

[27] B. Frieden, in Picture Processing and Digital Filtering, edited by T. Huang, Springer-Verlag,

Berlin (1975) 218.

[28] I. Cox, C. Sheppard, and T. Wilson, J. Opt. Soc. Am., 72 (1982) 1287-1291.

[29] V. Tamari (unpublished manuscript submitted to J. Opt. Soc. Am), (1985).

[30] M. Schaffner and G. Toraldo Di Francia, Nuovo Cimento, 6 (1949) 125.

[31] V. Tamari, U.S. Patent Appl. #007,971 (1987).

[32] C. Rushforth and R. Harris, J. Opt. Soc. Am., 58 (1968) 539-545.

[33] R. Luneberg, Mathematical Theory of Optics, Providence (1944) 391.

[34] G. Toraldo Di Francia, J. Opt. Soc. Am., 59 (1969) 799-804.

[35] N. Gridgeman, Math. Gazette, 54 (1970) 31-37.

[36] S. Goldstein, Modern Developments in Fluid Dynamics, Dover, New York, I (1965)41.

[37] V. Streeter and E. Wylie, Fluid Mechanics, McGraw Hill Int. ed., Singapore (1985).

[38] M. Gardner, Mathematical Carnival, Penguin Books, London (1975) 240.

[39] P. Jacquinot and B. Roizen-Dossier, Progress in Optics, edited by E. Wolf, 3,

North Holland (1964) 29.

[40] Kogelnik and Li, Applied Optics, 5 (1966) 1560.